nsablitz.blogg.se - 4 digit airfoil generator

Determine the final coordinates for the airfoil upper surface (xU, yU) and lower surface (xL, yL) using the following relationships.Calculate the thickness distribution above (+) and below (-) the mean line by plugging the value of t into the following equation for each of the x coordinates.

Compute the mean camber line coordinates by plugging the values of m and p into the following equations for each of the x coordinates.

Pick values of x from 0 to the maximum chord c.

Utilizing these m, p, and t values, we can compute the coordinates for an entire airfoil using the following relationships: For example, the NACA 2415 airfoil has a maximum thickness of 15% with a camber of 2% located 40% back from the airfoil leading edge (or 0.4c). The first digit specifies the maximum camber (m) in percentage of the chord (airfoil length), the second indicates the position of the maximum camber (p) in tenths of chord, and the last two numbers provide the maximum thickness (t) of the airfoil in percentage of chord. The first family of airfoils designed using this approach became known as the NACA Four-Digit Series. As airfoil design became more sophisticated, this basic approach was modified to include additional variables, but these two basic geometrical values remained at the heart of all NACA airfoil series, as illustrated below. They then presented a series of equations incorporating these two variables that could be used to generate an entire family of related airfoil shapes.

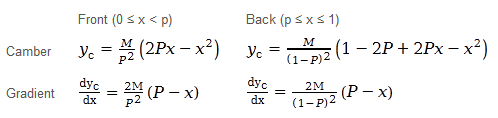
In this landmark report, the authors noted that there were many similarities between the airfoils that were most successful, and the two primary variables that affect those shapes are the slope of the airfoil mean camber line and the thickness distribution above and below this line. This methodology began to change in the early 1930s with the publishing of a NACA report entitled The Characteristics of 78 Related Airfoil Sections from Tests in the Variable Density Wind Tunnel. Before the National Advisory Committee for Aeronautics (NACA) developed these series, airfoil design was rather arbitrary with nothing to guide the designer except past experience with known shapes and experimentation with modifications to those shapes.

Later families, including the 6-Series, are more complicated shapes derived using theoretical rather than geometrical methods. As you suggest in your questions, the early NACA airfoil series, the 4-digit, 5-digit, and modified 4-/5-digit, were generated using analytical equations that describe the camber (curvature) of the mean-line (geometric centerline) of the airfoil section as well as the section's thickness distribution along the length of the airfoil.